by E.N. Tsyganov
(UA9 collaboration) University of Texas Southwestern
Medical Center at Dallas, Texas, USA
Abstract
Recent accelerator experiments on fusion of various elements have clearly demonstrated that the effective cross-sections of these reactions depend on what material the target particle is placed in. In these experiments, there was a significant increase in the probability of interaction when target nuclei are imbedded in a conducting crystal or are a part of it. These experiments open a new perspective on the problem of so-called cold nuclear fusion.
Recent accelerator experiments on fusion of various elements have clearly demonstrated that the effective cross-sections of these reactions depend on what material the target particle is placed in. In these experiments, there was a significant increase in the probability of interaction when target nuclei are imbedded in a conducting crystal or are a part of it. These experiments open a new perspective on the problem of so-called cold nuclear fusion.
PACS.: 25.45 – deuterium induced reactions
Submitted to Physics of Atomic Nuclei/Yadernaya Fizika in Russian
Introduction
Experiments of Fleischmann and Pons made about 20 years ago [1], raised the question about the possibility of nuclear DD fusion at room temperature. Conflicting results of numerous experiments that followed, dampened the initial euphoria, and the scientific community quickly came to common belief, that the results of [1] are erroneous. One of the convincing arguments of skeptics was the lack in these experiments of evidence of nuclear decay products. It was assumed that “if there are no neutrons, therefore is no fusion.” However, quite a large international group of physicists, currently a total of about 100-150 people, continues to work in this direction. To date, these enthusiasts have accumulated considerable experience in the field. The leading group of physicists working in this direction, in our opinion, is the group led by Dr. M. McKubre [2]. Interesting results were also obtained in the group of Dr. Y. Arata [3]. Despite some setbacks with the repeatability of results, these researchers still believe in the existence of the effect of cold fusion, even though they do not fully understand its nature. Some time ago we proposed a possible mechanism to explain the results of cold fusion of deuterium [4]. This work considered a possible mechanism of acceleration of deuterium contaminant atoms in the crystals through the interaction of atoms with long-wavelength lattice vibrations in deformed parts of the crystal. Estimates have shown that even if a very small portion of the impurity atoms (~105) get involved in this process and acquires a few keV energy, this will be sufficient to describe the energy released in experiments [2]. This work also hypothesized that the lifetime of the intermediate nucleus increases with decreasing energy of its excitation, so that so-called “radiation-less cooling” of the excited nucleus becomes possible. In [5], we set out a more detailed examination of the process. Quite recently, a sharp increase of the probability of fusion of various elements was found in accelerator experiments for the cases when the target particles are either imbedded in a metal crystal or are a part of the conducting crystal. These experiments compel us to look afresh on the problem of cold fusion.
Experiments of Fleischmann and Pons made about 20 years ago [1], raised the question about the possibility of nuclear DD fusion at room temperature. Conflicting results of numerous experiments that followed, dampened the initial euphoria, and the scientific community quickly came to common belief, that the results of [1] are erroneous. One of the convincing arguments of skeptics was the lack in these experiments of evidence of nuclear decay products. It was assumed that “if there are no neutrons, therefore is no fusion.” However, quite a large international group of physicists, currently a total of about 100-150 people, continues to work in this direction. To date, these enthusiasts have accumulated considerable experience in the field. The leading group of physicists working in this direction, in our opinion, is the group led by Dr. M. McKubre [2]. Interesting results were also obtained in the group of Dr. Y. Arata [3]. Despite some setbacks with the repeatability of results, these researchers still believe in the existence of the effect of cold fusion, even though they do not fully understand its nature. Some time ago we proposed a possible mechanism to explain the results of cold fusion of deuterium [4]. This work considered a possible mechanism of acceleration of deuterium contaminant atoms in the crystals through the interaction of atoms with long-wavelength lattice vibrations in deformed parts of the crystal. Estimates have shown that even if a very small portion of the impurity atoms (~105) get involved in this process and acquires a few keV energy, this will be sufficient to describe the energy released in experiments [2]. This work also hypothesized that the lifetime of the intermediate nucleus increases with decreasing energy of its excitation, so that so-called “radiation-less cooling” of the excited nucleus becomes possible. In [5], we set out a more detailed examination of the process. Quite recently, a sharp increase of the probability of fusion of various elements was found in accelerator experiments for the cases when the target particles are either imbedded in a metal crystal or are a part of the conducting crystal. These experiments compel us to look afresh on the problem of cold fusion.
Recent experiments on fusion of elements on accelerators
For atom-atom collisions the expression of the probability of penetration through a Coulomb barrier for bare nuclei should be modified, because atomic electrons screen the repulsion effect of nuclear charge. Such a modification for the isolated atom collisions has been performed in H.J. Assenbaum and others [6] using static Born-Oppenheimer approximation. The experimental results that shed further light on this problem were obtained in relatively recent works C. Rolfs [7] and K. Czerski [8]. Review of earlier studies on this subject is contained in the work of L. Bogdanova [9]. In these studies a somewhat unusual phenomenon was observed: the sub-barrier fusion cross sections of elements depend strongly on the physical state of the matter in which these processes are taking place. Figure 1 (left) shows the experimental data [8], demonstrating the dependence of the astrophysical factor S(E) for the fusion of elements of sub-threshold nuclear reaction on the aggregate state of the matter that contains the target nucleus 7Li. The same figure (right) presents similar data [7] for the DD reaction, when the target nucleus was embedded in a zirconium crystal. It must be noted that the physical nature of the phenomenon of increasing cross synthesis of elements in the case where this process occurs in the conductor crystal lattice is still not completely clear.
For atom-atom collisions the expression of the probability of penetration through a Coulomb barrier for bare nuclei should be modified, because atomic electrons screen the repulsion effect of nuclear charge. Such a modification for the isolated atom collisions has been performed in H.J. Assenbaum and others [6] using static Born-Oppenheimer approximation. The experimental results that shed further light on this problem were obtained in relatively recent works C. Rolfs [7] and K. Czerski [8]. Review of earlier studies on this subject is contained in the work of L. Bogdanova [9]. In these studies a somewhat unusual phenomenon was observed: the sub-barrier fusion cross sections of elements depend strongly on the physical state of the matter in which these processes are taking place. Figure 1 (left) shows the experimental data [8], demonstrating the dependence of the astrophysical factor S(E) for the fusion of elements of sub-threshold nuclear reaction on the aggregate state of the matter that contains the target nucleus 7Li. The same figure (right) presents similar data [7] for the DD reaction, when the target nucleus was embedded in a zirconium crystal. It must be noted that the physical nature of the phenomenon of increasing cross synthesis of elements in the case where this process occurs in the conductor crystal lattice is still not completely clear.
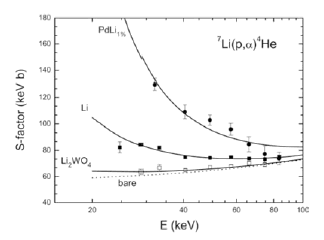
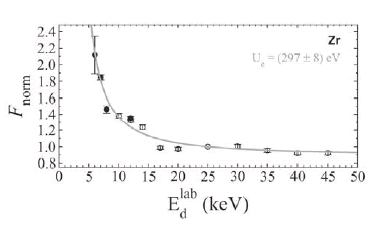Figure 1. Up – experimental data [8], showing the energy dependence of the S-factor for sub-threshold nuclear reaction on the aggregate state of matter that contains the nucleus 7Li. Down – the similar data [7] for the reaction of DD, when the target nucleus is placed in a crystal of zirconium. The data are well described by the introduction of the screening potential of about 300 eV.
The phenomenon is apparently due to the strong anisotropy of the electrical fields of the crystal lattice in the presence of free conduction electrons. Data for zirconium crystals for the DD reactions can be well described by the introduction of the screening potential of about 300 eV. It is natural to assume that the corresponding distance between of two atoms of deuterium in these circumstances is less than the molecular size of deuterium. In the case of the screening potential of 300 eV, the distance of convergence of deuterium atoms is ~510ˆ12 m, which is about an order of magnitude smaller than the size of a molecule of deuterium, where the screening potential is 27 eV. As it turned out, the reaction rate for DD fusion in these conditions is quite sufficient to describe the experimental results of McKubre and others [2]. Below we present the calculation of the rate process similar to the mu-catalysis where, instead of the exchange interaction by the muon, the factor of bringing together two deuterons is the effect of conduction electrons and the lattice of the crystal.
Calculation of the DD fusion rate for “Metal-Crystal” catalysis
The expression for the cross section of synthesis in the collision of two nuclei can be written as
The expression for the cross section of synthesis in the collision of two nuclei can be written as
where for the DD fusion
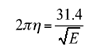Here the energy E is shown in keV in the center of mass. S(E) astrophysical factor (at low energies it can be considered constant), the factor 1/E reflects de Broglie dependence of cross section on energy. The main energy dependence of the fusion is contained in an expression
that determines the probability of penetration of the deuteron through the Coulomb barrier. From the above expressions, it is evident that in the case of DD collisions and in the case of DDμcatalysis, the physics of the processes is the same. We use this fact to determine the probability of DD fusion in the case of the “metal-crystalline” DD-catalysis. In the case of DDμ- catalysis the size of the muon deuterium molecules (ion+) is ~5×10ˆ13m. Deuterium nuclei approach such a distance at a kinetic energy ~3 keV. Using the expression (1), we found that the ratio of σ(3.0 keV)/σ(0.3 keV) = 1.05×10ˆ16. It should be noted that for the free deuterium molecule this ratio [ σ(3.0keV)/σ(0.03keV)] is about 10ˆ73. Experimental estimations of the fusion rate for the (DDμ)+ case presented in the paper by Hale [10]:
Thus, we obtain for the “metal-crystalline” catalysis DD fusion rate (for zirconium case):
Is this enough to explain the experiments on cold fusion? We suppose that a screening potential for palladium is about the same as for zirconium. 1 cmˆ3 (12.6 g) of palladium contains 6.0210ˆ23(12.6/106.4) = 0.710ˆ23 atoms. Fraction of crystalline cells with dual (or more) the number of deuterium atoms at a ratio of D: Pd ~1:1 is the case in the experiments [2] ~0.25 (e.g., for Poisson distribution). Crystal cell containing deuterium atoms 0 or 1, in the sense of a fusion reaction, we consider as “passive”. Thus, the number of “active” deuterium cells in 1 cmˆ3 of palladium is equal to 1.810ˆ22. In this case, in a 1 cmˆ3 of palladium the reaction rate will be
this corresponds to the energy release of about 3 kW. This is quite sufficient to explain the results of McKubre group [2]. Most promising version for practical applications would be Platinum (Pt) crystals, where the screening potential for d(d,p)t fusion at room temperature is about 675 eV [11]. In this case, DD fusion rate would be:
The problem of “nonradiative” release of nuclear fusion energy
As we have already noted, the virtual absence of conventional nuclear decay products of the compound nucleus was widely regarded as one of the paradoxes of DD fusion with the formation of 4He in the experiments [2]. We proposed the explanation of this paradox in [4]. We believe that after penetration through the Coulomb barrier at low energies and the materialization of the two deuterons in a potential well, these deuterons retain their identity for some time. This time defines the frequency of further nuclear reactions. Figure 2 schematically illustrates the mechanism of this process. After penetration into the compound nucleus at a very low energy, the deuterons happen to be in a quasi-stabile state seating in the opposite potential wells. In principle, this system is a dual “electromagnetic-nuclear” oscillator. In this oscillator the total kinetic energy of the deuteron turns into potential energy of the oscillator, and vice versa. In the case of very low-energy, the amplitude of oscillations is small, and the reactions with nucleon exchange are suppressed.
As we have already noted, the virtual absence of conventional nuclear decay products of the compound nucleus was widely regarded as one of the paradoxes of DD fusion with the formation of 4He in the experiments [2]. We proposed the explanation of this paradox in [4]. We believe that after penetration through the Coulomb barrier at low energies and the materialization of the two deuterons in a potential well, these deuterons retain their identity for some time. This time defines the frequency of further nuclear reactions. Figure 2 schematically illustrates the mechanism of this process. After penetration into the compound nucleus at a very low energy, the deuterons happen to be in a quasi-stabile state seating in the opposite potential wells. In principle, this system is a dual “electromagnetic-nuclear” oscillator. In this oscillator the total kinetic energy of the deuteron turns into potential energy of the oscillator, and vice versa. In the case of very low-energy, the amplitude of oscillations is small, and the reactions with nucleon exchange are suppressed.
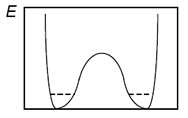Fig. 2. Schematic illustration of the mechanism of the nuclear decay frequency dependence on the compound nucleus 4He* excitation energy for the merging deuterons is presented. The diagram illustrates the shape of the potential well of the compound nucleus. The edges of the potential well are defined by the strong interaction, the dependence at short distances Coulomb repulsion.
The lifetime of the excited 4He* nucleus can be considered in the formalism of the usual radioactive decay. In this case,
Here ν is the decay frequency, i.e., the reciprocal of the decay time τ. According to our hypothesis, the decay rate is a function of excitation energy of the compound nucleus E. Approximating with the first two terms of the polynomial expansion, we have:
Here ν° is the decay frequency at asymptotically low excitation energy. According to quantum-mechanical considerations, the wave functions of deuterons do not completely disappear with decreasing energy, as illustrated by the introduction of the term ν°. The second term of the expansion describes the linear dependence of the frequency decay on the excitation energy. The characteristic nuclear frequency is usually about 10ˆ22 sˆ-1. In fusion reaction D+D4He there is a broad resonance at an energy around 8 MeV. Simple estimates by the width of the resonance and the uncertainty relation gives a lifetime of the intermediate state of about 0.810ˆ22 s. The “nuclear” reaction rate falls approximately linearly with decreasing energy. Apparently, a group of McKubre [2] operates in an effective energy range below 2 keV in the c.m.s. Thus, in these experiments, the excitation energy is at least 4×10ˆ3 times less than in the resonance region. We assume that the rate of nuclear decay is that many times smaller. The corresponding lifetime is less than 0.3×10ˆ18 s. This fall in the nuclear reaction rate has little effect on the ratio of output decay channels of the compound nucleus, but down to a certain limit. This limit is about 6 keV. A compound nucleus at this energy is no longer an isolated system, since virtual photons from the 4He* can reach to the nearest electron and carry the excitation energy of the compound nucleus. The total angular momentum carried by the virtual photons can be zero, so this process is not prohibited. For the distance to the nearest electron, we chose the radius of the electrons in the helium atom (3.1×10ˆ11 m). From the uncertainty relations, duration of this process is about 10ˆ-19 seconds. In the case of “metal-crystalline” catalysis the distance to the nearest electrons can be significantly less and the process of dissipation of energy will go faster. It is assumed that after an exchange of multiple virtual photons with the electrons of the environment the relatively small excitation energy of compound nucleus 4He* vanishes, and the frequency of the compound nucleus decaying with the emission of nucleons will be determined only by the term ν°. For convenience, we assume that this value is no more than 10ˆ12-10ˆ14 per second. In this case, the serial exchange of virtual photons with the electrons of the environment in a time of about 10ˆ-16 will lead to the loss of ~4 MeV from the compound nucleus (after which decays with emission of nucleons are energetically forbidden), and then additional exchange will lead to the loss of all of the free energy of the compound nucleus (24 MeV) and finally the nucleus will be in the 4He ground state. The energy dissipation mechanism of the compound nucleus 4He* with virtual photons, discussed above, naturally raises the question of the electromagnetic-nuclear structure of the excited compound nucleus.
Fig. 3. Possible energy diagram of the excited 4He* nucleus is presented.
Figure 3 represents a possible energy structure of the excited 4He* nucleus and changes of its spatial configuration in the process of releasing of excitation energy. Investigation of this process might be useful to study the quark-gluon dynamics and the structure of the nucleus.
Discussion
Perhaps, in this long-standing history of cold fusion, finally the mystery of this curious and enigmatic phenomenon is gradually being opened. Besides possible benefits that the practical application of this discovery will bring, the scientific community should take into account the sociological lessons that we have gained during such a long ordeal of rejection of this brilliant, though largely accidental, scientific discovery. We would like to express the special appreciation to the scientists that actively resisted the negative verdict imposed about twenty years ago on this topic by the vast majority of nuclear physicists.
Perhaps, in this long-standing history of cold fusion, finally the mystery of this curious and enigmatic phenomenon is gradually being opened. Besides possible benefits that the practical application of this discovery will bring, the scientific community should take into account the sociological lessons that we have gained during such a long ordeal of rejection of this brilliant, though largely accidental, scientific discovery. We would like to express the special appreciation to the scientists that actively resisted the negative verdict imposed about twenty years ago on this topic by the vast majority of nuclear physicists.
Acknowledgements
The author thanks Prof. S.B. Dabagov, Dr. M. McKubre, Dr. F. Tanzela, Dr. V.A. Kuzmin, Prof. L.N. Bogdanova and Prof. T.V. Tetereva for help and valuable discussions. The author is grateful to Prof. V.G. Kadyshevsky, Prof. V.A. Rubakov, Prof. S.S. Gershtein, Prof. V.V. Belyaev, Prof. N.E. Tyurin, Prof. V.L. Aksenov, Prof. V.M. Samsonov, Prof. I.M. Gramenitsky, Prof. A.G. Olshevsky, Prof. V.G. Baryshevsky for their help and useful advice. I am grateful to Dr. VM. Golovatyuk, Prof. M.D. Bavizhev, Dr. N.I. Zimin, Prof. A.M. Taratin for their continued support. I am also grateful to Prof. A. Tollestrup, Prof. U. Amaldi, Prof. W. Scandale, Prof. A. Seiden, Prof. R. Carrigan, Prof. A. Korol, Prof. J. Hauptmann, Prof. V. Guidi, Prof. F. Sauli, Prof. G. Mitselmakher, Prof. A. Takahashi, and Prof. X. Artru for stimulating feedback. Continued support in this process was provided with my colleagues and the leadership of the University of Texas Southwestern Medical Center at Dallas, and I am especially grateful to Prof. R. Parkey, Prof. N. Rofsky, Prof. J. Anderson and Prof. G. Arbique. I express special thanks to my wife, N.A. Tsyganova for her stimulating ideas and uncompromising support.
The author thanks Prof. S.B. Dabagov, Dr. M. McKubre, Dr. F. Tanzela, Dr. V.A. Kuzmin, Prof. L.N. Bogdanova and Prof. T.V. Tetereva for help and valuable discussions. The author is grateful to Prof. V.G. Kadyshevsky, Prof. V.A. Rubakov, Prof. S.S. Gershtein, Prof. V.V. Belyaev, Prof. N.E. Tyurin, Prof. V.L. Aksenov, Prof. V.M. Samsonov, Prof. I.M. Gramenitsky, Prof. A.G. Olshevsky, Prof. V.G. Baryshevsky for their help and useful advice. I am grateful to Dr. VM. Golovatyuk, Prof. M.D. Bavizhev, Dr. N.I. Zimin, Prof. A.M. Taratin for their continued support. I am also grateful to Prof. A. Tollestrup, Prof. U. Amaldi, Prof. W. Scandale, Prof. A. Seiden, Prof. R. Carrigan, Prof. A. Korol, Prof. J. Hauptmann, Prof. V. Guidi, Prof. F. Sauli, Prof. G. Mitselmakher, Prof. A. Takahashi, and Prof. X. Artru for stimulating feedback. Continued support in this process was provided with my colleagues and the leadership of the University of Texas Southwestern Medical Center at Dallas, and I am especially grateful to Prof. R. Parkey, Prof. N. Rofsky, Prof. J. Anderson and Prof. G. Arbique. I express special thanks to my wife, N.A. Tsyganova for her stimulating ideas and uncompromising support.
References
1. M. Fleischmann, S. Pons, M. W. Anderson, L. J. Li, M. Hawkins, J. Electro anal. Chem. 287, 293 (1990).
2. M. C. H. McKubre, F. Tanzella, P. Tripodi, and P. Haglestein, In Proceedings of the 8th International Conference on Cold Fusion. 2000, Lerici (La Spezia), Ed. F. Scaramuzzi, (Italian Physical Society, Bologna, Italy, 2001), p 3; M. C. H. McKubre, In Condensed Matter Nuclear Science: Proceedings Of The 10th International Conference On Cold Fusion; Cambridge, Massachusetts, USA 21-29 August, 2003, Ed by P. L. Hagelstein and S. R. Chubb, (World Sci., Singapore, 2006). M. C. H. McKubre, “Review of experimental measurements involving dd reactions”, Presented at the Short Course on LENR for ICCF-10, August 25, 2003.
3. Y. Arata, Y. Zhang, “The special report on research project for creation of new energy”, J. High Temp. Soc. (1) (2008).
4. E. Tsyganov, in Physics of Atomic Nuclei, 2010, Vol. 73, No. 12, pp. 1981–1989. Original Russian text published in Yadernaya Fizika, 2010, Vol. 73, No. 12, pp. 2036–2044.
5. E.N. Tsyganov, “The mechanism of DD fusion in crystals”, submitted to IL NUOVO CIMENTO 34 (4-5) (2011), in Proceedings of the International Conference Channeling 2010 in Ferrara, Italy, October 3-8 2010.
6. H.J. Assenbaum, K. Langanke and C. Rolfs, Z. Phys. A – Atomic Nuclei 327, p. 461-468 (1987).
7. C. Rolfs, “Enhanced Electron Screening in Metals: A Plasma of the Poor Man”, Nuclear Physics News, Vol. 16, No. 2, 2006.
8. A. Huke, K. Czerski, P. Heide, G. Ruprecht, N. Targosz, and W. Zebrowski, “Enhancement of deuteron-fusion reactions in metals and experimental implications”, PHYSICAL REVIEW C 78, 015803 (2008).
9. L.N. Bogdanova, Proceedings of International Conference on Muon Catalyzed Fusion and Related Topics, Dubna, June 18–21, 2007, published by JINR, E4, 15-2008-70, p. 285-293
10. G.M. Hale, “Nuclear physics of the muon catalyzed d+d reactions”, Muon Catalyzed Fusion 5/6 (1990/91) p. 227-232.
11. F. Raiola (for the LUNA Collaboration), B. Burchard, Z. Fulop, et al., J. Phys. G: Nucl. Part. Phys.31, 1141 (2005); Eur. Phys. J. A 27, s01, 79 (2006).
1. M. Fleischmann, S. Pons, M. W. Anderson, L. J. Li, M. Hawkins, J. Electro anal. Chem. 287, 293 (1990).
2. M. C. H. McKubre, F. Tanzella, P. Tripodi, and P. Haglestein, In Proceedings of the 8th International Conference on Cold Fusion. 2000, Lerici (La Spezia), Ed. F. Scaramuzzi, (Italian Physical Society, Bologna, Italy, 2001), p 3; M. C. H. McKubre, In Condensed Matter Nuclear Science: Proceedings Of The 10th International Conference On Cold Fusion; Cambridge, Massachusetts, USA 21-29 August, 2003, Ed by P. L. Hagelstein and S. R. Chubb, (World Sci., Singapore, 2006). M. C. H. McKubre, “Review of experimental measurements involving dd reactions”, Presented at the Short Course on LENR for ICCF-10, August 25, 2003.
3. Y. Arata, Y. Zhang, “The special report on research project for creation of new energy”, J. High Temp. Soc. (1) (2008).
4. E. Tsyganov, in Physics of Atomic Nuclei, 2010, Vol. 73, No. 12, pp. 1981–1989. Original Russian text published in Yadernaya Fizika, 2010, Vol. 73, No. 12, pp. 2036–2044.
5. E.N. Tsyganov, “The mechanism of DD fusion in crystals”, submitted to IL NUOVO CIMENTO 34 (4-5) (2011), in Proceedings of the International Conference Channeling 2010 in Ferrara, Italy, October 3-8 2010.
6. H.J. Assenbaum, K. Langanke and C. Rolfs, Z. Phys. A – Atomic Nuclei 327, p. 461-468 (1987).
7. C. Rolfs, “Enhanced Electron Screening in Metals: A Plasma of the Poor Man”, Nuclear Physics News, Vol. 16, No. 2, 2006.
8. A. Huke, K. Czerski, P. Heide, G. Ruprecht, N. Targosz, and W. Zebrowski, “Enhancement of deuteron-fusion reactions in metals and experimental implications”, PHYSICAL REVIEW C 78, 015803 (2008).
9. L.N. Bogdanova, Proceedings of International Conference on Muon Catalyzed Fusion and Related Topics, Dubna, June 18–21, 2007, published by JINR, E4, 15-2008-70, p. 285-293
10. G.M. Hale, “Nuclear physics of the muon catalyzed d+d reactions”, Muon Catalyzed Fusion 5/6 (1990/91) p. 227-232.
11. F. Raiola (for the LUNA Collaboration), B. Burchard, Z. Fulop, et al., J. Phys. G: Nucl. Part. Phys.31, 1141 (2005); Eur. Phys. J. A 27, s01, 79 (2006).
by E.N. Tsyganov
(UA9 collaboration) University of Texas Southwestern
Medical Center at Dallas, Texas, USA
(UA9 collaboration) University of Texas Southwestern
Medical Center at Dallas, Texas, USA
